5.4.2 Leaching

     The leaching process is assumed to occur by advective transport of the aqueous contaminant out of the bottom face of the source zone along with the percolating vadose zone water. This means that the rate of loss of mass at any given time is given by the volumetric flux of water out of the source zone face multiplied by the aqueous concentration of the contaminant in the water at that time ^(a)



The volumetric water flux, in turn, can be expressed in terms of the Darcy water flux density (i.e., Darcy velocity) of the percolating water and the area of the bottom face of the source zone:



By substituting Equation 5.59 into Equation 5.58, the mass flux equation for loss from the source zone by leaching alone can be expressed as



     When a NAPL phase exists in the source zone, the aqueous concentration of the contaminant is controlled by the composition of the NAPL phase. In this case, the source-term release module calculates the value of C_wiin Equation 5.60 by the phase partitioning theory described in Section 2.2.4 (which uses different methods, depending on the mole fraction [or concentration] of the contaminant in the NAPL phase).

     When no NAPL phase exists in the source zone, the aqueous concentration can be calculated by a simple phase partitioning relation (i.e., Equation 2.7 in Section 2.2.3). When leaching is the only loss process occurring, the vertical thickness, (z_b-z_t), of the source zone is constant in time. In this case, the volume of the source zone is given by



where

z_b is the distance from the initial position of the soil surface to the bottom of the source zone at any time (cm)
z_t is the distance from the initial position of the soil surface to the initial position of the top of the source zone (i.e., initial thickness of clean layer) (cm).

Substituting Equation 5.61 into Equation 2.7, the aqueous concentration can be given by



Now, substituting Equation 5.62 into Equation 5.60, the mass flux equation for loss from the source zone by leaching (when no NAPL is present) can be expressed as



     Recall that the retardation factor, R_i, in Equation 5.63 was defined in general terms by Equation 2.5. It is worthwhile to note that for the contaminated vadose zone source zone, the solid-sorbent bulk density in Equation 2.5 is equal to the soil bulk density, ß_s.

     The above equations were for leaching from contaminant source zones that have not been subjected to remediation methodologies. However, they are also appropriate to scenarios where a remediation methodology has been implemented that only alters the source zone by changing the value of some parameter in the aforementioned model (e.g., reducing the contaminant inventory, reducing the water flux through the zone, increasing the sorption coefficient).

     Two additional remediation methodologies implemented in the source-term release module require special theory (i.e., formulations that differ from the mass flux expressions for unremediated scenarios), because leaching occurs by fundamentally different mechanisms than those described by the previous equations. The first additional remediation methodology is ISV, which is a methodology that consists of converting the contaminant source zone into a glass waste form. The resulting glass waste form is a cracked (rather than a solid) monolith, with much internal surface area exposed to percolating vadose-zone water. Contaminants are released as the glass slowly dissolves into the percolating water from all of its exposed surface area. The second additional remediation methodology is ISS, which is a methodology that consists of injecting liquid grout material into the pores of the source zone and allowing it to solidify into a solid microporous monolith. Contaminants are released as they slowly diffuse through the grout to the outer surface boundary of the monolith, where they first encounter water percolating down through the vadose zone.

  For times after an ISV remediation methodology has been implemented, the contaminant release mechanism is dissolution of the cracked glass waste form. The glass waste form is assumed to be a so-called "well-mixed reactor." Therefore, the mass flux equation for loss from the source zone by leaching alone for an ISV waste form is given by



where

r_g is the mass dissolution rate of glass in an ISV waste form per surface area (g yr^-1 cm^-2)
A_sg is the total surface area of cracked glass in an ISV waste form (cm²)
r_g is the density of glass in an ISV waste form (g cm^-3)
V_g is the volume of cracked glass in an ISV waste form (cm³).

     Note that A_sg and V_g would not be constant in real-world situations, but instead would be changing over time as the glass dissolves. This means that to be accurate, Equation 5.64 would need to be further developed by substituting into it appropriate expressions for how the surface area and volume change as a function of time. In general, these expressions would depend on the initial shape and size of the waste form, and how it is assumed to crack over time. For all but simple initial shapes and cracking patterns, it would be extremely difficult, if not impossible, to derive analytical expressions for A_sg(t) and V_g(t). Therefore, the equation implemented in the module assumes the following idealization. When leaching is the only loss process occurring, the overall total concentration of a contaminant in the glass is always given by its initial value:



where

M_io' is the total mass or activity of contaminant i in the source zone at the time the zone was subjected to ISV remediation (g or Ci)
V_go is the initial volume of cracked glass in an ISV waste form (cm³).

 If it is assumed that the surface area of the glass is always equal to its initial value, the mass flux equation for loss from the source zone by leaching alone for an ISV waste form is given by



where A_sgo is the initial total surface area of cracked glass in an ISV waste form (cm²).

 This idealization implicitly assumes that the decrease in surface area caused by the dissolving glass pieces shrinking is just equal to the increase in surface area caused by additional glass cracking.

     Note further that when a site is remediated with an ISV process, all the organic contaminants are vaporized during the vitrification process and no longer exist in the resulting ISV waste form. Therefore, simulations with organic contaminants and an ISV waste form present from time zero should not be conducted. If the simulated scenario contains a baseline period before an ISV process is implemented, the masses of all organic contaminants should be zero for all times after implementation of the ISV.

     For times after an ISS remediation methodology has been implemented, the contaminant release mechanism is diffusion through the grout within the source zone to the outer boundary of the solidified waste form. Therefore, the mass flux equation for loss from the source zone by leaching alone for an ISS waste form is given by



where

M_io" is the total mass or activity of contaminant i in the source zone at the time the zone was subjected to ISS remediation (g or Ci)
D_gi is the effective diffusion coefficient of contaminant i within an ISS waste form (cm² yr^-1).

     This equation is the solution to the diffusion equation for mass lost through an infinite plane that bounds a semi-infinite solid source, when no degradation/decay occurs (Godbee et al. 1980). The mass flux calculated by Equation 5.67 is approximately equal to that coming from a finite solid source for early times. However, at later times, Equation 5.67 will overpredict the mass flux by an increasing amount as time goes on. Note also that Equation 5.67 depends on the mass in the source zone at the time when the ISS methodology was implemented in the zone (i.e., when grout was injected into the zone), rather than on the mass in the source zone at any given time (as in the previous mass flux equations). This arises from the fact that the conceptual model used here requires that a spatial gradient in concentration be present within the waste form. This is in direct opposition to the "well-mixed reactor" assumption used to derive mass flux expressions in all of the other scenarios dealt with. This contradiction will cause some restrictions regarding additional loss routes that can be accommodated when formulating expressions for mass flux for multiple, concurrent loss pathway scenarios. Furthermore, because Equation 5.67 depends on M_io" rather than M_i, partial failure of an ISS waste form cannot be simulated (i.e., simulating partial failure cannot be done by merely adjusting some parameter in Equation 5.67). However, total failure of an ISS waste form at some designated time can be simulated. In this case the leaching term reverts back to Equation 5.60 or 5.63 at the time of failure; and the source zone begins to be treated as a "well-mixed reactor" again (with mass equal to the mass remaining in the ISS waste form when it failed). In addition, this dependence on M_io" (rather than M_i) also means that mass cannot be added to the source zone during a simulation through chain decay from parent contaminants. Additional special theory is used to accommodate daughter product accumulation and loss; but this is discussed in the section on multiple concurrent loss processes.

     In certain environments (e.g., where the vadose zone water flux density is very low), Equations 5.66 and 5.67 may predict greater leaching loss fluxes than the equations for unremediated source zones (i.e., Equations 5.60 or 5.63). This is not realistic; and happens only because Equations 5.66 and 5.67 assume that the rate-limiting process is the release from the waste form. What is actually happening in the real environment is that movement in the soil water would be the rate-limiting step. Hence, the module compares mass fluxes predicted by the equations for remediated and unremediated scenarios and uses the smaller loss flux.

---

 

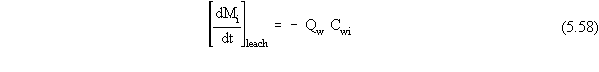
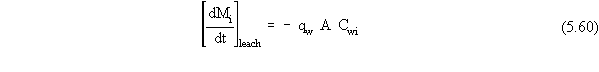
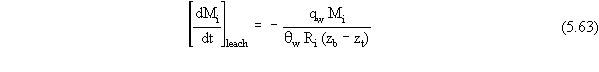
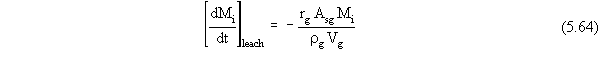
(a)

Note that, in a strict sense, the variable Qw should really be referred to as the aqueous solution flux (which contains water and dissolved constituents). However, because aqueous solutions are typically volumetrically dilute with respect to their dissolved constituents, it is quite common to refer to the aqueous solution flux as the water flux.