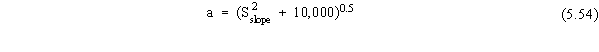5.3.3 Slope Length and Steepness Factor
The slope length and steepness factor (LS-factor)
describes the combined effects of slope length (i.e., flow length) and
slope gradient (i.e., grade or relief); it represents the ratio of soil
loss per unit area on a site to the corresponding loss from a 22.1-m- (72.6-ft-)
long experimental plot with a 9% slope. Slope length is defined as the
distance from the point of origin of overland flow to the point where the
slope decreases sufficiently for deposition to occur or to the point where
runoff enters a defined channel (wet or dry). The slope steepness is the
segment or site slope, usually expressed as a percentage. Although the
LS-factor has traditionally been expressed as two parameters in the USLE,
it is universally computed as a combined term (Mitchell and Bubenzer 1980;
Goldman et al. 1986); which is why Equation 5.49 is written with the single
factor Sfact.
Slope length and slope steepness strongly
influence the transport of soil particles once the soil particles are dislodged
by raindrop impact or runoff. Because the LS-factor can be defined to be
substantially greater than unity, it can have a considerable effect on
the predicted erosion. For this reason, averaging over large areas is not
advised. In fact, Foster et al. (1980) suggest not basing the LS-factor
solely on United States Geological Survey (USGS) topographic maps, as they
usually suggest excessively long slope lengths.
Goldman et al. (1986) have mathematically expressed the LS-factor as follows:

in which
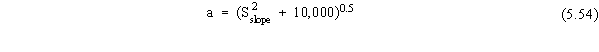
where
Sslope is the slope gradient (in percent) (unitless)
a is a factor in the LS-factor equation (unitless)
Lslope is the slope length (cm)
mLS is the exponent in the LS-factor equation (unitless).
The exponent, mLS, is equal to: 0.2 for Sslope
< 1%; 0.3 for 1% Sslope 3%; 0.4 for 3.5% Sslope
4.5%; 0.5 for Sslope 5%. Equations 5.53 and 5.54 have been expressed
as a figure by a number of authors (e.g., Shultz et al. 1986; Novotny and
Chesters 1981; Mitchell and Bubenzer 1980; Wischmeier and Smith 1978).