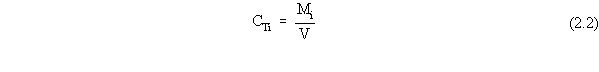
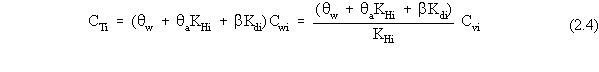
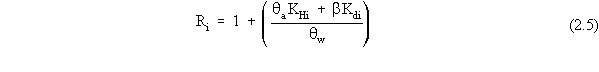
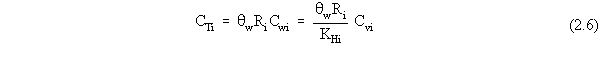

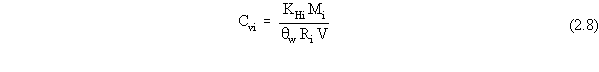
| (a) | In these scenarios, ß is more explicitly defined as ßs, the soil bulk density. In addition, for a contaminated aquifer, the volumetric air content, qa, is zero, and the volumetric water content, qw, is more explicitly defined as the total porosity, qt. |
| (b) | In this scenario, ß is more explicitly defined as ßss, the suspended sediment concentration. |